Simple Harmonic Motion

The $\mathrm{x}-\mathrm{t}$ graph of a particle undergoing simple harmonic motion is as shown in the figure.

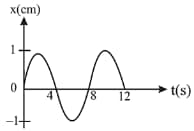
The acceleration of the particle at $\mathrm{t}=\frac{4}{3}\mathrm{ }\mathrm{s}$ is

Two particles are performing SHM in same phase. It means that:
 

If two SHMs of different amplitudes are added together, the resultant SHM will be a maximum if the phase difference between them is:

Two particles execute simple harmonic motions of same amplitude and frequency along the same straight line. They cross one another when going opposite directions. The phase difference between them when their displacements are one half of their amplitudes is then:

In simple harmonic motion, potential energy value at extreme position is _____.

In simple harmonic motion, At the extreme position kinetic energy is zero.

Which one of this will be zero at extreme position of simple harmonic motion?

When two particles executing SHM are overlapped in opposite phase then resultant amplitude will be 

 A is the amplitude, ω is the angular frequency, and φ is called the _____.

The displacement of two particles executing SHM are expressed as ${\mathrm{y}}_1=\mathrm{a} \sin \left(\mathrm{\pi t}+\mathrm{\varphi }\right)$ and ${\mathrm{y}}_2=\mathrm{b} \sin \left(2\mathrm{\pi t}+\mathrm{\varphi }\right)$ then at $\mathrm{t}=1 \sec$ both are

When two SHMS are super imposed  so that resultant amplitude becomes minimum then both are overlapped in _____ phase?

What is initial phase of oscillation?

Two simple harmonic motions have displacements ${\mathrm{x}}_1=12 \sin \left(\mathrm{\omega t}+\raisebox{1ex}{$\mathrm{\pi }$}\!\left/ \!\raisebox{-1ex}{$2$}\right.\right)$ and ${\mathrm{x}}_2=6 \sin \left(\mathrm{\omega t}+\raisebox{1ex}{$3\mathrm{\pi }$}\!\left/ \!\raisebox{-1ex}{$2$}\right.\right)$ are superimposed on each other. Resulting amplitude will be.

The Initial phase in SHM is $y=a \sin \left(\omega t+\varphi \right)$.

What is the initial phase in Simple Harmonic Motion ?

Thus, simple harmonic motion (SHM) is not any periodic motion but one in which displacement is sinusoidal function of time.

Two SHM’s are respectively by $y=a_1\sin (\omega t-kx)$ and $y=a_2\cos (\omega t-kx).$ The phase difference between the two is

When a spring-mass system vibrates with simple harmonic motion, the mass in motion reaches its maximum velocity when its acceleration is _____ (maximum\minimum).

A block of mass $\mathrm{m}=4 \mathrm{kg}$ undergoes simple harmonic motion with amplitude$\mathrm{A}= 6 \mathrm{cm}$ on the frictionless surface. Block is attached to a spring of force constant $k=400 \mathrm{N} {\mathrm{m}}^{-1}$ . If the block is at$\mathrm{x}=6 \mathrm{cm}$ at time $t=0$ and equilibrium position is at $x=0$ then the blocks position as a function of time (with x in centimetres and t in seconds) ?

A mass of $36 \mathrm{kg}$ is kept vertically on the top of a massless spring. What is the maximum compression of the spring if the spring constant is $15000 \mathrm{N}/\mathrm{m}$. Assume $\mathrm{g} = 10\mathrm{m}/{\mathrm{s}}^2$.